Strongly correlated many body system is fantasy world for searching exotic quantum phases, including but not limited to spin liquid, high-Tc superconductivity and topological phase transitions. In order to understand the physical mechanism, we using numerical method (especially quantum Monte Carlo simulations) to study various quantum many body systems, including frustrated magnetism, cold atoms in optical lattice, cavity-QED and Rydberg array.

2022.06.08 Quantum dynamics of topological strings in a frustrated Ising antiferromagnet

Zheng Zhou, Changle Liu, Zheng Yan*, Yan Chen*, and Xue-Feng Zhang*

We investigate the quantum dynamics of the antiferromagnetic transverse field Ising model on the triangular lattice through largescale quantum Monte Carlo simulations and stochastic analytic continuation. This model effectively describes a series of triangular 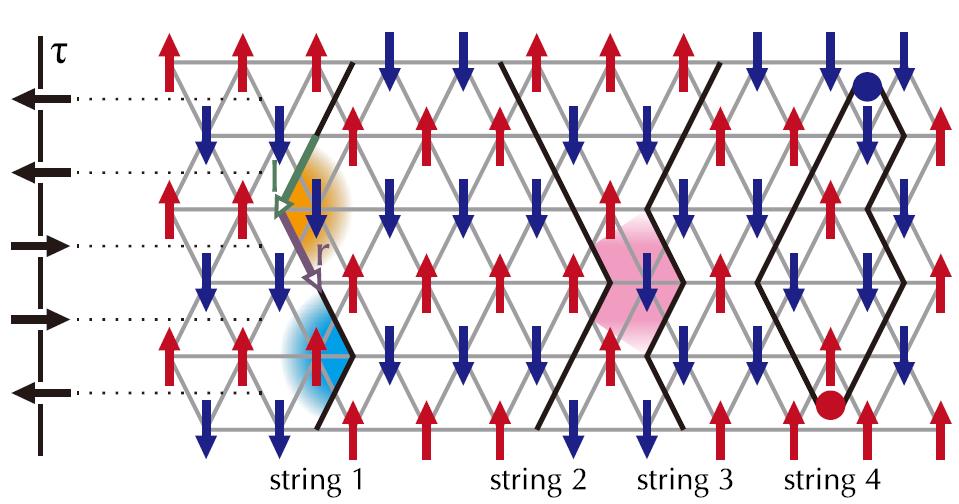rare-earth compounds, for example, TmMgGaO4. At weak transverse field, we capture the excitations related to topological quantum strings, which exhibit continuum features described by XY chain along the strings and those in accord with 'Luttinger string liquid' in the perpendicular direction. The continuum features can be well understood from the perspective of topological strings. Furthermore, we identify the contribution of strings from the excitation spectrum. Our study provides characteristic features for the experimental search for string-related excitations and proposes a theoretical method to pinpoint topological excitations in the experimental spectra.

NPJ Quantum Materials 7: 60 (2022)

2022.02.17 Floquet Engineering Hz-Level Rabi Spectra in Shallow Optical Lattice Clock

Mo-Juan Yin, Xiao-Tong Lu, Ting Li, Jing-Jing Xia, Tao Wang, Xue-Feng Zhang*, and Hong Chang*

Quantum metrology with ultrahigh precision usually requires atoms prepared in an ultrastable environment with well-defined quantum states. Thus, in optical lattice clock systems deep lattice potentials are used to trap ultracold atoms. However, decoherence, induced by Raman scattering and higher order light shifts, can significantly be reduced if atomic clocks are realized in shallow optical lattices. On the other hand, in such lattices, tunneling among different sites can cause additional dephasing and strongly broadening of the Rabi spectrum. Here, in our experiment, we periodically drive a shallow 87Sr optical lattice clock. Counterintuitively, shaking the system can deform the wide broad spectral line into a sharp peak with 5.4 Hz linewidth. With careful comparison between the theory and experiment, we demonstrate that the Rabi frequency and the Bloch bands can be tuned, simultaneously and independently. Our work not only provides a different idea for quantum metrology, such as building shallow optical lattice clock in outer space, but also paves the way for quantum simulation of new phases of matter by engineering exotic spin orbit couplings.

Phys. Rev. Lett. 128, 073603 (2022)

2021.08.02 Quantum Phases in a Quantum Rabi Triangle

Yu-Yu Zhang, Zi-Xiang Hu, Libin Fu, Hong-Gang Luo, Han Pu, and Xue-Feng Zhang*

The interplay of interactions, symmetries, and gauge fields usually leads to intriguing quantum manybody phases. To explore the nature of emerging phases, we study a quantum Rabi triangle system as an elementary building block for synthesizing an artificial magnetic field. We develop an analytical approach to study the rich phase diagram and the associated quantum criticality. Of particular interest is the emergence of a chiral-coherent phase, which breaks both the Z2 and the chiral symmetry. In this chiral phase, photons flow unidirectionally and the chirality can be tuned by the artificial gauge field, exhibiting a signature of broken time-reversal symmetry. The finite-frequency scaling analysis further confirms the associated phase transition to be in the universality class of the Dicke model. This model can simulate a broad range of physical phenomena of light-matter coupling systems, and may have an application in future developments of various quantum information technologies.

Phys. Rev. Lett. 127, 063602 (2021)

2021.06.16 Doubly Modulated Optical Lattice Clock: Interference and Topology

Xiao-Tong Lu, Tao Wang, Ting Li, Chi-Hua Zhou, Mo-Juan Yin, Ye-Bing Wang, Xue-Feng Zhang*, and Hong Chang*

The quantum system under periodical modulation is the simplest path to understand the quantum nonequilibrium system because it can be well described by the effective static Floquet Hamiltonian. Under the stroboscopic measurement, the initial phase is usually irrelevant. However, if two uncorrelated parameters are modulated, their relative phase cannot be gauged out so that the physics can be dramatically changed. 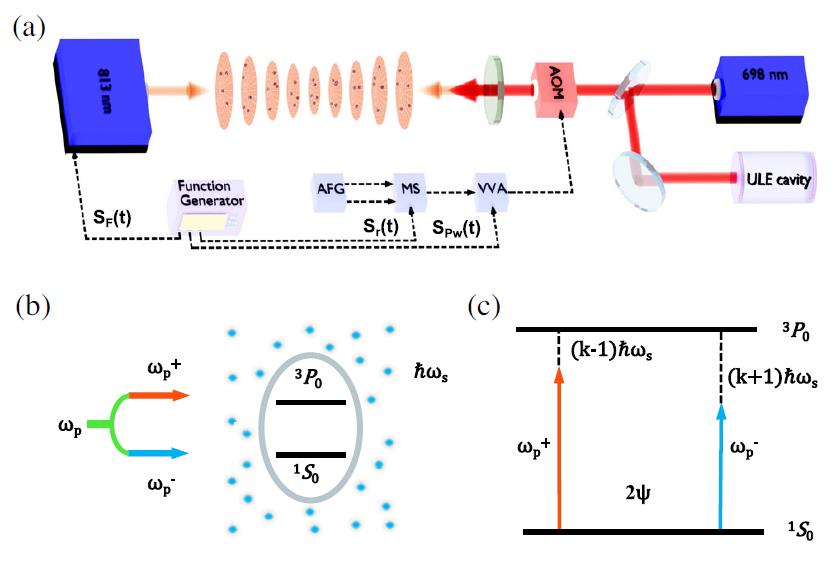Here, we simultaneously modulate the frequency of the lattice laser and the Rabi frequency in an optical lattice clock (OLC) system. Thanks to the ultrahigh precision and ultrastability of the OLC, the relative phase could be fine-tuned. As a smoking gun, we observed the interference between two Floquet channels. Finally, by experimentally detecting the eigenenergies, we demonstrate the relation between the effective Floquet Hamiltonian and the one-dimensional topological insulator with a high winding number. Our experiment not only provides a direction for detecting the phase effect but also paves a way in simulating the quantum topological phase in the OLC platform.

Phys. Rev. Lett. 127, 033601 (2021)

2019.03.08 Machine learning of quantum phase transitions

Xiao-Yu Dong, Frank Pollmann, and Xue-Feng Zhang*

Machine learning algorithms provide a new perspective on the study of physical phenomena. In this Rapid Communication, we explore the nature of quantum phase transitions using a multicolor convolutional neural network (CNN) in combination with quantum Monte Carlo simulations. We propose a method that compresses 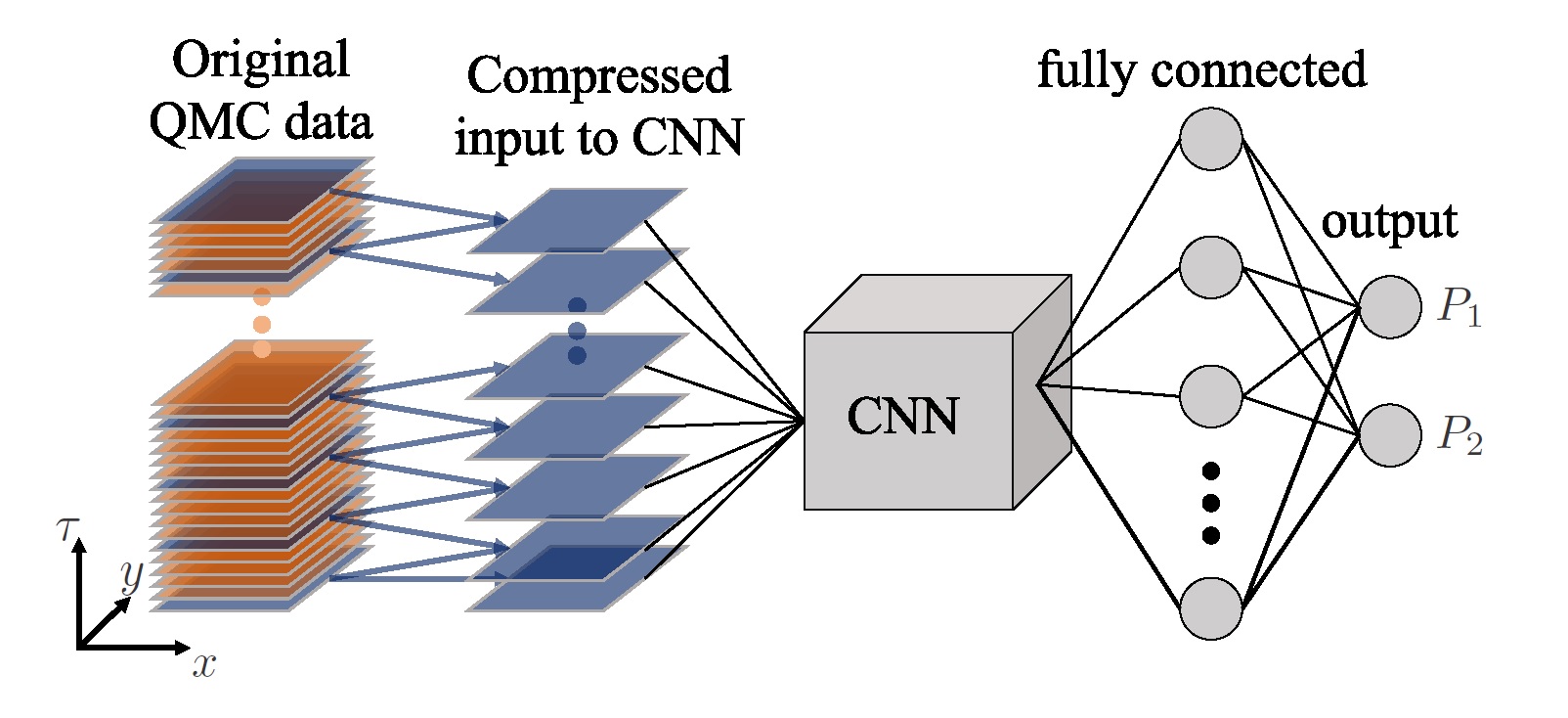(d + 1)-dimensional space-time configurations to a manageable size and then use them as the input for a CNN. We benchmark our approach on two models and show that both continuous and discontinuous quantum phase transitions can be well detected and characterized. Moreover, we show that intermediate phases, which were not trained, can also be identified using our approach

Phys. Rev. B 99, 121104(R) (2019)

2018.08.02 Quantum Spin Liquid with Even Ising Gauge Field Structure on Kagome Lattice

Yan-Cheng Wang, Xue-Feng Zhang*, Frank Pollmann, Meng Cheng, and Zi Yang Meng*

Employing large-scale quantum Monte Carlo simulations, we study the extended XXZ model on the kagome lattice. A Z2 quantum spin liquid phase with effective even Ising gauge field structure emerges 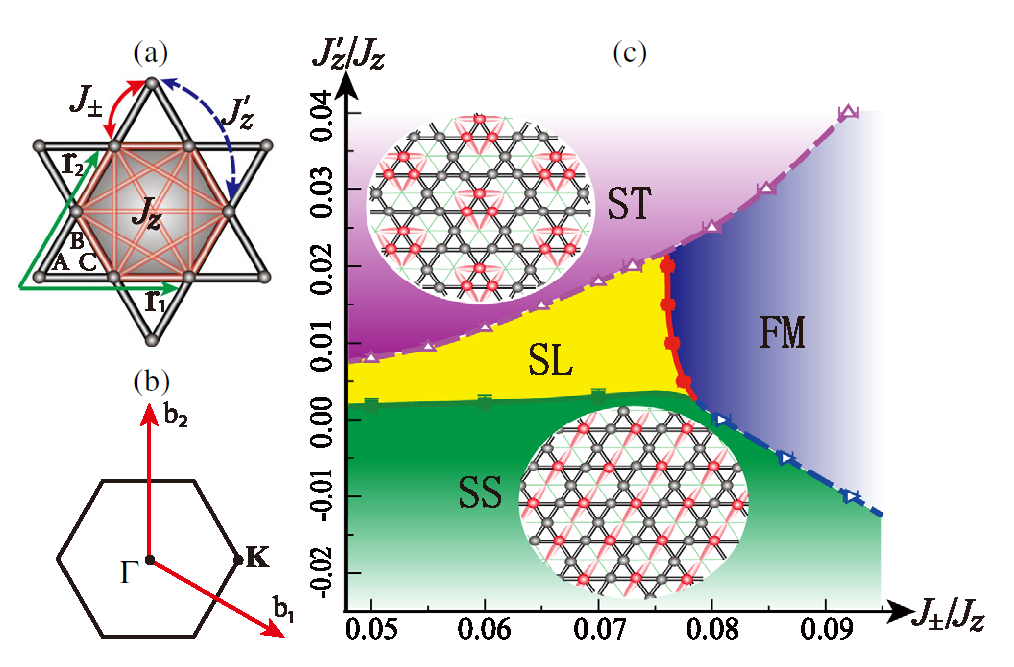from the delicate balance among three symmetry-breaking phases including stripe solid, staggered solid, and ferromagnet. This Z2 spin liquid is stabilized by an extended interaction related to the Rokhsar- Kivelson potential in the quantum dimer model limit. The phase transitions from the staggered solid to a spin liquid or ferromagnet are found to be first order and so is the transition between the stripe solid and ferromagnet. However, the transition between a spin liquid and ferromagnet is found to be continuous and belongs to the 3D XY* universality class associated with the condensation of spinons. The transition between a spin liquid and stripe solid appears to be continuous and associated with the condensation of visons.

Phys. Rev. Lett. 121, 057202 (2018)

2018.03.15 Continuous Easy-Plane Deconfined Phase Transition on the Kagome Lattice

Xue-Feng Zhang, Yin-Chen He, Sebastian Eggert, Roderich Moessner, and Frank Pollmann

We use large scale quantum Monte Carlo simulations to study an extended Hubbard model of hard core bosons on the kagome lattice. In the limit of strong nearest-neighbor interactions at 1/3 filling, the interplay between frustration and quantum fluctuations leads to a valence bond solid ground state. The system undergoes a quantum phase transition to a superfluid phase as the interaction strength is decreased. It is still under debate whether the transition is weakly first order or represents an unconventional continuous phase transition. We present a theory in terms of an easy plane noncompact CP1 gauge theory describing the phase transition at 1/3 filling. Utilizing large scale quantum Monte Carlo simulations with parallel tempering in the canonical ensemble up to 15552 spins, we provide evidence that the phase transition is continuous at exactly 1/3 filling. A careful finite size scaling analysis reveals an unconventional scaling behavior hinting at deconfined quantum criticality.

Phys. Rev. Lett. 120, 115702 (2018)

2016.11.03 Quantum Domain Walls Induce Incommensurate Supersolid Phase on the Anisotropic Triangular Lattice

Xue-Feng Zhang*, Shijie Hu*, Axel Pelster, and Sebastian Eggert

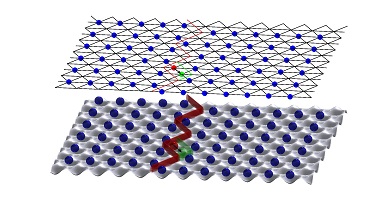
We investigate the extended hard-core Bose-Hubbard model on the triangular lattice as a function of spatial anisotropy with respect to both hopping and nearest-neighbor interaction strength. At half-filling the system can be tuned from decoupled one-dimensional chains to a two-dimensional solid phase with alternating density order by adjusting the anisotropic coupling. At intermediate anisotropy, however, frustration effects dominate and an incommensurate supersolid phase emerges, which is characterized by incommensurate density order as well as an anisotropic superfluid density. We demonstrate that this intermediate phase results from the proliferation of topological defects in the form of quantum bosonic domain walls. Accordingly, the structure factor has peaks at wave vectors, which are linearly related to the number of domain walls in a finite system in agreement with extensive quantum Monte Carlo simulations. We discuss possible connections with the supersolid behavior in the high-temperature superconducting striped phase.

Phys. Rev. Lett. 117, 193201 (2016)

2015.02.09 The phase diagram of the antiferromagnetic XXZ model on the triangular lattice (Editor's Suggestion)

Daniel Sellmann, Xue-Feng Zhang*, and Sebastian Eggert

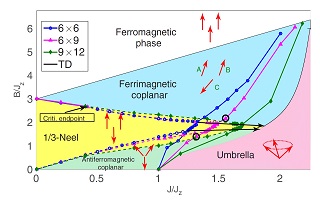
We determine the quantum phase diagram of the antiferromagnetic spin-1/2 XXZ model on the triangular lattice as a function of magnetic field and anisotropic coupling Jz. Using the density matrix renormalization group algorithm in two dimensions, we establish the locations of the phase boundaries between a plateau phase with 1/3 Neel order and two distinct coplanar phases. The two coplanar phases are characterized by a simultaneous breaking of both translational and U(1) symmetries, which is reminiscent of supersolidity. A translationally invariant umbrella phase is entered via a first-order phase transition at relatively small values of Jz compared to the corresponding case of ferromagnetic hopping and the classical model. The phase transition lines meet at two tricritical points on the tip of the lobe of the plateau state, so that the two coplanar states are completely disconnected. Interestingly, the phase transition between the plateau state and the upper coplanar state changes from second order to first order for large values of Jz larger than 2.5J.

Phys. Rev. B 91, 081104(R) (2015)

2014.11.14 Phase diagram of the triangular extended Hubbard model

Luca F. Tocchio, Claudius Gros, Xue-Feng Zhang, and Sebastian Eggert

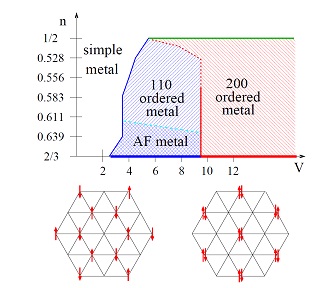
We study the extended Hubbard model on the triangular lattice as a function of filling and interaction strength. The complex interplay of kinetic frustration and strong interactions on the triangular lattice leads to exotic phases where long-range charge order, antiferromagnetic order, and metallic conductivity can coexist. Variational Monte Carlo simulations show that three kinds of ordered metallic states are stable as a function of nearest neighbor interaction and filling. The coexistence of conductivity and order is explained by a separation into two functional classes of particles: part of them contributes to the stable order, while the other part forms a partially filled band on the remaining substructure. The relation to charge ordering in charge transfer salts is discussed.

Phys. Rev. Lett. 113, 246405 (2014)

2013.09.30 Chiral Edge States and Fractional Charge Separation in a System of Interacting Bosons on a Kagome Lattice

Xue-Feng Zhang and Sebastian Eggert

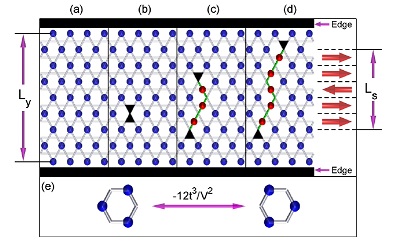
We consider the extended hard-core Bose-Hubbard model on a kagome lattice with boundary conditions on two edges. We find that the sharp edges lift the degeneracy and freeze the system into a striped order at 1/3 and 2/3 filling for zero hopping. At small hopping strengths, holes spontaneously appear and separate into fractional charges which move to the edges of the system. This leads to a novel 1 edge liquid phase, which is characterized by fractional charges near the edges and a finite edge compressibility but no superfluid density. The compressibility is due to excitations on the edge which display a chiral symmetry breaking that is reminiscent of the quantum Hall effect and topological insulators. Large scale Monte Carlo simulations confirm the analytical considerations.

Phys. Rev. Lett. 111, 147201 (2013)

2013.02.28 Rydberg Polaritons in a Cavity: A Superradiant Solid (Editor's suggestion)

Xue-Feng Zhang, Qing Sun, Yu-Chuan Wen, Wu-Ming Liu, Sebastian Eggert, and An-Chun Ji

We study an optical cavity coupled to a lattice of Rydberg atoms, which can be represented by a generalized Dicke model. We show that the competition between the atom-atom interaction and atomlight coupling induces a rich phase diagram. A novel superradiant solid (SRS) phase is found, where both the superradiance and crystalline orders coexist. Different from the normal second order superradiance transition, here both the solid-1=2 and SRS to SR phase transitions are first order. These results are confirmed by large scale quantum Monte Carlo simulations.

Phys. Rev. Lett. 110, 090402 (2013)